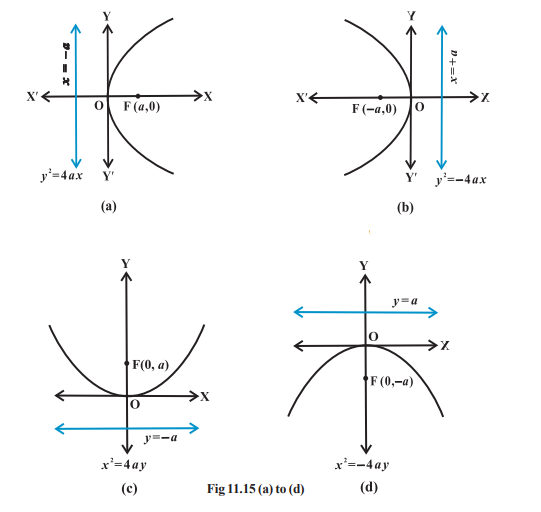
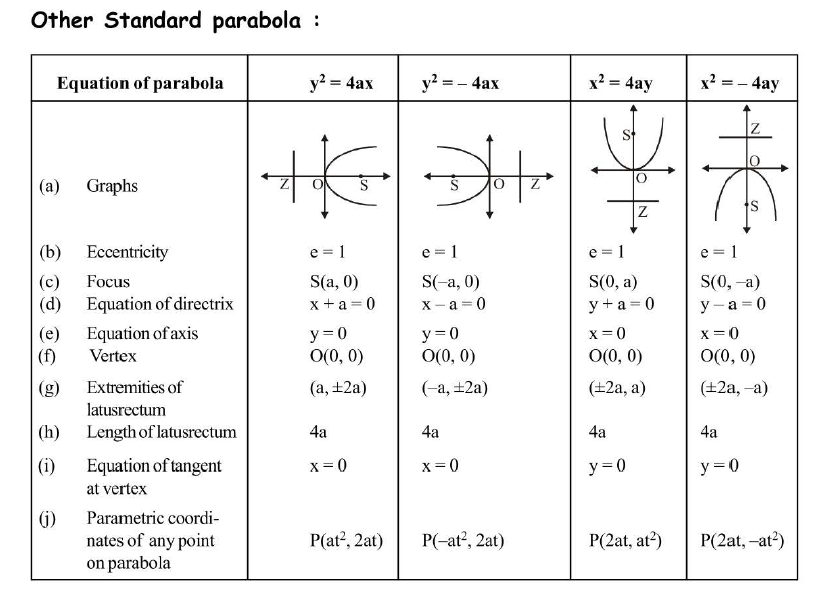
`\color{green} ✍️` The equation of a parabola is simplest if the vertex is at the origin and the axis of symmetry is along the `x-`axis or `y-`axis. The four possible such orientations of parabola are shown below in`Fig \ \ 11.15` `(a)` to `(d).`
`\color{green} "We will derive the equation for the parabola shown above in Fig 11.15 (a)"` with focus at `(a, 0) a > 0;` and directricx `x = – a` as below:
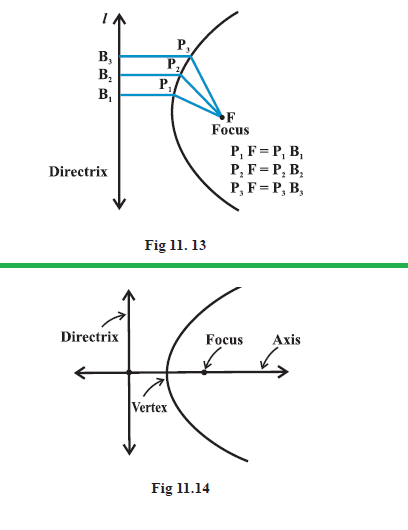
Let `F` be the focus and `l` the directrix. Let FM be perpendicular to the directrix and bisect FM at the point `O`. Produce `MO` to `X`.
By the definition of parabola, the mid-point `O` is on the parabola and is called the vertex of the parabola.
Take `O` as origin, OX the x-axis and `OY` perpendicular to it as the `y-`axis.
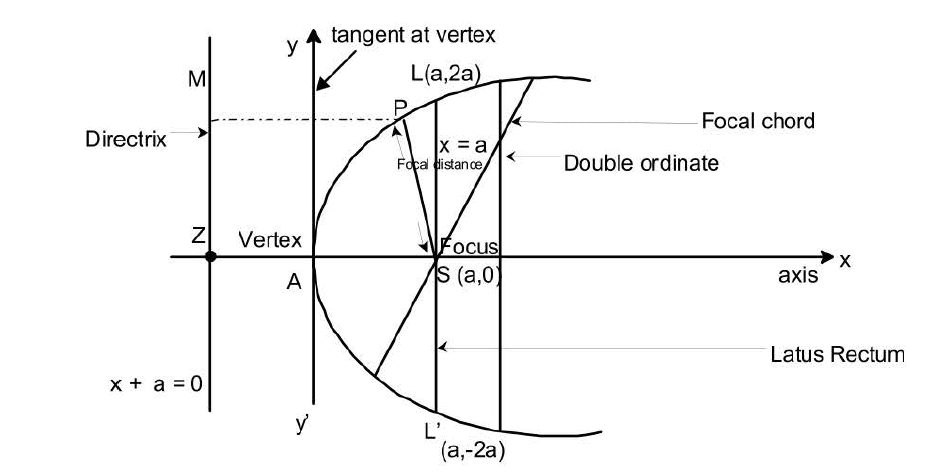
Let the distance from the directrix to the focus be `2a.` Then, the coordinates of the focus are `(a, 0)`, and the equation of the directrix is `x + a = 0` as in Fig11.16. Let `P(x, y)` be any point on the parabola such that `PF = PB,`
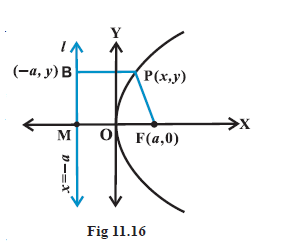
where `PB` is perpendicular to `l`. The coordinates of `B` are `(– a, y).` By the distance formula, we have
`PF = sqrt((x-a)^2 +y^2)` and `PB = sqrt((x+a)^2`
Since `PF = PB,` we have
`sqrt((x-a)^2 +y^2) = sqrt((x+a)^2`
i.e. `(x – a)^2 + y^2 = (x + a)^2`
or `x^2 - 2ax + a^2 + y^2 = x^2 + 2ax + a^2`
or `y^2 = 4ax ( a > 0).`
Hence, any point on the parabola satisfies
`y^2 = 4ax.`
Conversely, let `P(x, y)` satisfy the equation (2)
`PF = sqrt((x-a)^2 +y^2) = sqrt((x-a)^2 + 4ax)`
`= sqrt[(x+a)^2] = PB`
and so `P(x,y)` lies on the parabola.
Thus, from (2) and (3) we have proved that the equation to the parabola with vertex at the origin, focus at (a,0) and directrix `x = – a` is `y^2 = 4ax.`
Discussion In equation `(2),` since `a > 0, x` can assume any positive value or zero but no negative value and the curve extends indefinitely far into the first and the fourth quadrants. The axis of the parabola is the positive x-axis. Similarly, we can derive the equations of the parabolas in:
Fig 11.15 (b) as `y^2 = – 4ax,`
Fig 11.15 (c) as `x^2 = 4ay,`
Fig 11.15 (d) as `x^2 = – 4ay,`
These four equations are known as `color{green}"standard equations of parabolas."`
`color{blue} "☛Note :"` The standard equations of parabolas have focus on one of the coordinate axis; vertex at the origin and thereby the directrix is parallel to the other coordinate axis. However, the study of the equations of parabolas with focus at any point and any line as directrix is beyond the scope here.
`\color{green} ✍️` The equation of a parabola is simplest if the vertex is at the origin and the axis of symmetry is along the `x-`axis or `y-`axis. The four possible such orientations of parabola are shown below in`Fig \ \ 11.15` `(a)` to `(d).`
`\color{green} "We will derive the equation for the parabola shown above in Fig 11.15 (a)"` with focus at `(a, 0) a > 0;` and directricx `x = – a` as below:
Let `F` be the focus and `l` the directrix. Let FM be perpendicular to the directrix and bisect FM at the point `O`. Produce `MO` to `X`.
By the definition of parabola, the mid-point `O` is on the parabola and is called the vertex of the parabola.
Take `O` as origin, OX the x-axis and `OY` perpendicular to it as the `y-`axis.
Let the distance from the directrix to the focus be `2a.` Then, the coordinates of the focus are `(a, 0)`, and the equation of the directrix is `x + a = 0` as in Fig11.16. Let `P(x, y)` be any point on the parabola such that `PF = PB,`

where `PB` is perpendicular to `l`. The coordinates of `B` are `(– a, y).` By the distance formula, we have
`PF = sqrt((x-a)^2 +y^2)` and `PB = sqrt((x+a)^2`
Since `PF = PB,` we have
`sqrt((x-a)^2 +y^2) = sqrt((x+a)^2`
i.e. `(x – a)^2 + y^2 = (x + a)^2`
or `x^2 - 2ax + a^2 + y^2 = x^2 + 2ax + a^2`
or `y^2 = 4ax ( a > 0).`
Hence, any point on the parabola satisfies
`y^2 = 4ax.`
Conversely, let `P(x, y)` satisfy the equation (2)
`PF = sqrt((x-a)^2 +y^2) = sqrt((x-a)^2 + 4ax)`
`= sqrt[(x+a)^2] = PB`
and so `P(x,y)` lies on the parabola.
Thus, from (2) and (3) we have proved that the equation to the parabola with vertex at the origin, focus at (a,0) and directrix `x = – a` is `y^2 = 4ax.`
Discussion In equation `(2),` since `a > 0, x` can assume any positive value or zero but no negative value and the curve extends indefinitely far into the first and the fourth quadrants. The axis of the parabola is the positive x-axis. Similarly, we can derive the equations of the parabolas in:
Fig 11.15 (b) as `y^2 = – 4ax,`
Fig 11.15 (c) as `x^2 = 4ay,`
Fig 11.15 (d) as `x^2 = – 4ay,`
These four equations are known as `color{green}"standard equations of parabolas."`
`color{blue} "☛Note :"` The standard equations of parabolas have focus on one of the coordinate axis; vertex at the origin and thereby the directrix is parallel to the other coordinate axis. However, the study of the equations of parabolas with focus at any point and any line as directrix is beyond the scope here.